Quantum Materials

Designing materials for hosting quantum skyrmions

Background

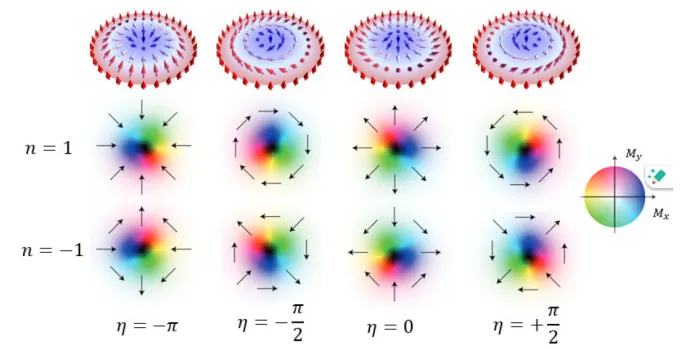
Since the first observation of magnetic skyrmions in MnSi¹⁰⁸, a non-centrosymmetric material with strong DMI, most of the reported experimental results on skyrmions have focused on materials with strong DMI. A strong bulk DMI can stabilize Bloch-type skyrmions in non-centrosymmetric materials, e.g., MnSi, FeGe¹⁰⁹ and Cu₂OSeO₃ ^18,110, while Néel-type skyrmions can readily form in ferromagnetic/heavy metal (FM/HM) layered thin films with interfacial DMI^{15,32,33,111,112}. The DMI is determined by the spin-orbit coupling under the broken inversion symmetry, and its dependence on crystal or interface structure locks magnetic skyrmions to a certain helicity angle η^113–115 (FIG. 3-1).

Unlike traditional magnetic skyrmions stabilized by strong DMI, the magnetic skyrmions required for helicity-based skyrmion qubits^21,116 are formed in material systems with DMI = 0, allowing the additional internal degree of freedom^17,20 associated with the rotation of the helicity. For the quantum nature of the skyrmions to be revealed, the size of the skyrmions should be sufficiently small, less than 10 nm, which we refer to as nanoskyrmions in this proposal. Additionally, ultralow Gilbert damping (α~10^-5 to 10^-4 ) is desired for candidate materials to ensure sufficiently long coherence times²². The Gilbert damping constant is closely related to the coherence time, which is a crucial parameter in quantum computing that measures how long a qubit can maintain its quantum state without being disturbed by its environment. The lower the Gilbert damping constant, the longer the coherence time. The theoretically proposed helicity-based skyrmion qubit is estimated to have a coherence time in the microsecond regime by using a material with ultralow Gilbert damping α = 10⁻⁵ at 100 mK.

Encoding quantum information in helicity-based skyrmion qubits

Therefore, to encode quantum information in helicity-based skyrmion qubits as proposed by theoreticians, we need to search for magnetic materials that fulfill three criteria:

1. the capability of hosting nanoskyrmions
2. absent or negligible DMI to allow the helicity degree of freedom
3. ultralow Gilbert damping for long qubit lifetime.

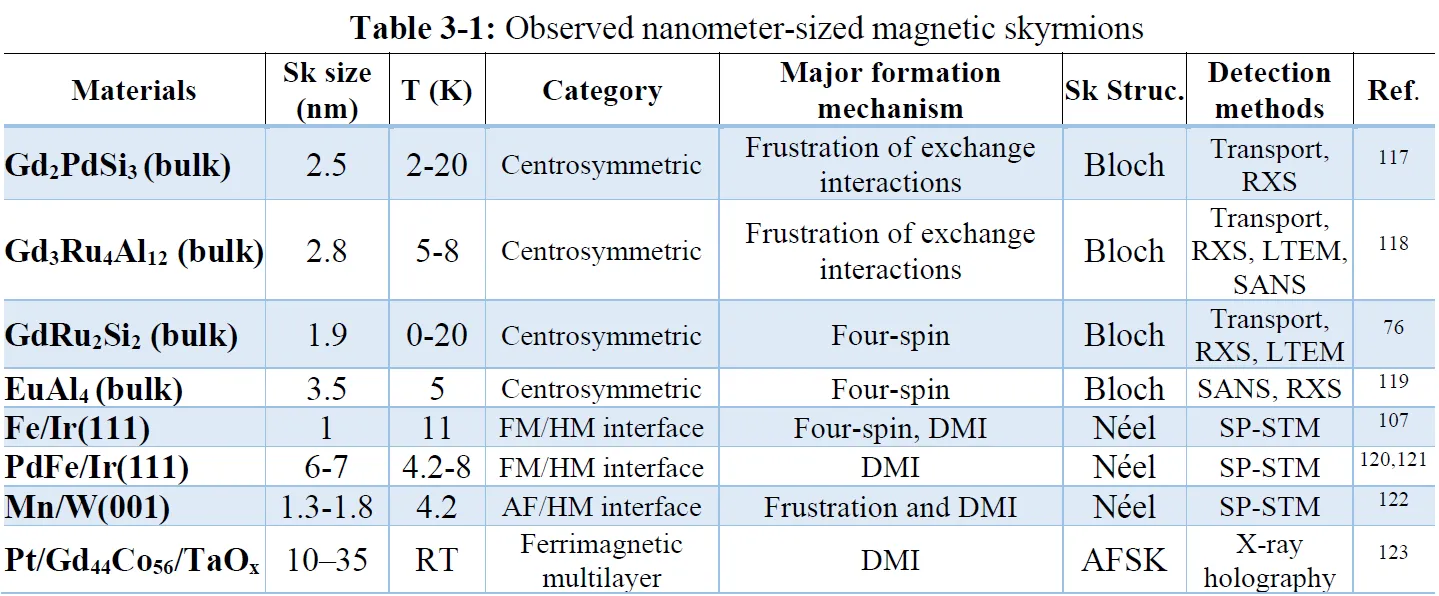
We summarize the nanoskyrmions observed by various experimental methods as well as the major skyrmion formation mechanisms in Table 3-1. Nanoskyrmions mainly form in two types of materials: centrosymmetric crystals (FIG. 3-2a) and interfaces or multilayers with interfacial DMI (FIG. 3-2c).

Skyrmion formation results from the interplay of various magnetic interactions in the materials, such as the Heisenberg exchange interactions, long-range magnetic dipolar interactions^23,63,114, Zeeman energy, magnetic anisotropy, DMI, four-spin interactions^76,107, and coupling between itinerant electron spins and localized spins^124,125. For example, FIG. 3-2d shows the major interactions involved in the formation of the square array of nanoskyrmions at the hexagonal Fe monolayer (ML) on the Ir (111) surface. The interplay of the Heisenberg exchange, four-spin, and DMI is the microscopic origin of this observed nanoskyrmion state¹⁰⁷.

Nanoskyrmions stabilized by the interfacial DMI have a fixed helicity determined by the sign of DMI, so they cannot be used for helicity-based skyrmion qubits. In centrosymmetric magnets, DMI is absent due to the intrinsic inversion symmetry, the origin of nanoskyrmion formation^17,115 in these materials involves the frustration of exchange interactions²⁰ and four-spin interactions mediated by itinerant electrons in the presence of easy-axis anisotropy^76,107. The energies of these skyrmions are degenerate with respect to the helicity degree of freedom if the magnetic dipolar interaction is neglected. When the magnetic dipolar interaction is significant, the helicity is locked to two-fold degenerate Bloch-type skyrmions by the dipolar interaction^21,63,126 (FIG. 3-2b), which can be used for coding quantum information. Understanding these skyrmion formation mechanisms can shed light on the design and search for new materials that host nanoskyrmions.

However, the experimental results on the Gilbert damping constant for the centrosymmetric materials in Table 3-1 are lacking, which is not surprising given the challenges of growing high-quality samples for these materials as well as the large magnetic field and low temperature required for the skyrmion phase. Reported magnetic thin films with measured ultralow Gilbert damping of the order of α ∼ 10⁻⁴ − 10⁻⁵ include insulating oxides with perpendicular magnetic anisotropy (PMA), such as yttrium iron garnet(YIG)^127,128 and Bi-doped YIG (BiYIG)¹²⁹ as well as half-metal magnetic Heusler compounds¹³⁰. There was also progress in obtaining α as low as 2.1 × 10⁻³ in sputtered 10 nm-thick polycrystalline Co₂₅Fe₇₅ alloys¹³¹.

Investigation of the Gilbert damping of candidate materials is important for developing materials that allow observation of the quantum nature of the skyrmions. Several theoretical approaches¹³¹ have been developed to predict magnetic damping in metallic systems, which include Kambersky's breathing Fermi-surface model^132–134, the generalized torque correlation model by Gilmore et al. 135, and Thonig et al. 136, a scattering theory-based model by Brataas et al.137 and Liu et al.138, and a numerical realization of a linear response damping model implemented by Mankovsky¹³⁹.

We are comparing these models and identifying suitable models to predict the Gilbert damping constant of our candidate materials and further estimate the coherence times of the skyrmion qubits²².

Centrosymmetric magnets

Inspired by the discovered materials that can host nanoskyrmions (Table 3-1), we have started our search of candidate materials for skyrmion qubits from the family of centrosymmetric magnets with frustrated exchange interactions or four-spin interactions, including Gd₂PdSi₃, Gd₃Ru₄Al₁₂, GdRu₂Si₂, RGa₂ (R: rare earth element) with the same space group as Gd₂PdSi₃, such as TbGa₂. Additional materials  considered²² include NiGa₂S₄, Fe₃Sn₂, MnInP₂Te₆, α-NaFeO₂, and SrFeO₃.

Self-intercalated covalent 2D magnets

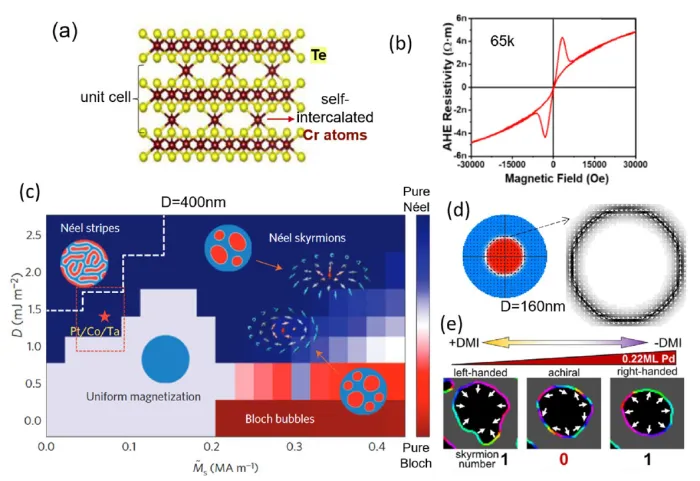
In addition to searching for bulk materials that can host nanoskyrmions, we are exploring self-intercalated covalent 2D magnets with a general chemical formula M_1+δX₂, such as Cr₂Te₃ and Cr₅Te₈ ^78,140. In such a material, native 3d transition metal atoms M (such as Cr, Mn, Fe, Co and Ni) are self-intercalated in the vdW gap between a bilayer of transition metal dichalcogenide MX₂ (X is a chalcogen element such as S, Se or Te) in situ to form ultrathin, covalently bonded layered magnets (FIG. 3-3a).

PI Cheng and Dr. Hao Zeng from University at Buffalo have observed humps and dips in the hysteresis loops of Hall resistivity of a Cr₂Te₃ 2D layer (FIG. 3-3b), which may arise from the non-collinear spin textures, possible magnetic skyrmions, enabled by the competing exchange interactions.

Bloch skyrmions in nanodisks with zero DMI

Compared to bulk and thin films, the role of the magnetic dipolar interaction in nanodisks due to the shape anisotropy will become increasingly significant as the disk shrinks (FIG. 3-3c,d) ^141,142. Simulation results for a 160nm-diameter disk with perpendicular magnetic anisotropy (PMA) but absent DMI show that the interplay of the Heisenberg and dipolar interactions will result in a Bloch-type skyrmion (FIG. 3-3d) ¹⁴¹. Such Bloch skyrmions in nanodisks with zero DMI should be two-fold degenerate (𝜂𝜂 = ±𝜋𝜋/2).

We are fabricating nanodisks of YIG and BiYIG with PMA as well as Co_xFe_1−x alloy due to their ultralow Gilbert damping. We are also fabricating nanodisks of HM₁ capping/ epitaxial ultrathin magnetic layer on a single crystalline HM2 substrate, where negligible DMI is achieved by tuning the thickness of the HM₁ capping layer. Recently, Chen et al. have demonstrated the tuning of DMI across 0, revealed by spin-polarized low-energy electron microscopy (SP-LEEM) imaging of the evolution of skyrmion structures in (Ni/Co)₂/Pd(2.05 ML)/W(110) capped by Pd of various thicknesses¹¹³ (FIG. 3-3e). It is worth noting that the achiral domain wall of the bubble as the DMI crosses 0 is related to the micron-scale size of the bubble, which differs significantly from the nanodisk case where the dipolar interaction plays a significant role.

Suitable material systems for quantum skyrmions

To identify suitable material systems for quantum skyrmions (nm skyrmion size), Co-PI Buchanan is leading the modeling team to explore the proposed using the methods described earlier in Section 2.2.

1. centrosymmetric magnets
2. self-intercalated covalent 2D magnets
3. nanodisks with zero DMI

We have started with the material parameters reported in the references or obtained from first-principles calculations by Dr. Yin. The obtained modeling results are guiding material synthesis and device fabrication, as described in Section 3.1.2. The experimental results are, in turn, providing valuable feedback to improve our modeling, forming a closed loop for material and sample design. We are investigating the interplay of the relevant interactions in candidate materials as well as the effect of film thickness and disk size to determine the skyrmion size, shape, vorticity, helicity, and stability.